Branchenbezogene Anwendungsbeispiele des Wolfram SystemModelers
Develop realistic models and digital twins for any industry with System Modeler. Core built-in components and custom libraries can represent elements and processes in any domain. Download a trial to experiment with the dynamic models yourself.
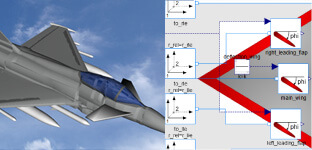
Aerospace & Defense
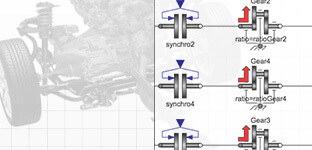
Automotive & Transportation
Heavy Equipment
Industrial Manufacturing
Consumer Products
Energy
Life Sciences
Business
Other
Aerospace & Defense
Model and test complex systems such as unmanned aerial vehicles (UAV), space robotics, and aircraft dynamics. SystemModeler enables system- and component-level design and continuous testing and verification.
Aircraft Catapult
Model a hydraulic aircraft catapult to accelerate an aircraft to a high speed in a short distance.
See ExampleFighter
Create a library for aircraft modeling, which can be used to study flight dynamics during different flight conditions.
See ExampleSatellite
Model a satellite with a combination of 3D mechanics and block-based control logic and visualize the path for the satellite.
See ExampleFlap System Reliability: Cessna 441
Compute the reliability of a Cessna 441 flap system using lifetime data for the individual hydraulic parts.
See ExampleAutomotive & Transportation
Design and simulate vehicle dynamics, powertrain controllers, chassis and safety systems, and more with SystemModeler. Combine with Mathematica for control system design and optimization.
Cruise control
Import an FMU of a cruise control model from Simulink and evaluate its performance in a car model in Wolfram SystemModeler.
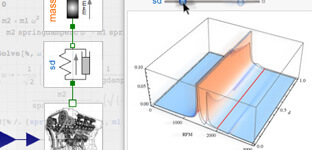
See ExampleDriveline
This example explores how the transmission control unit parameters affect fuel consumption for a midsize car.
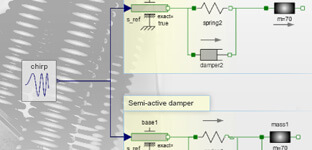
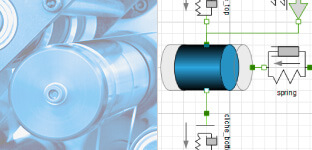
See ExampleTruck Seat Suspension
Model an active damper and compare with a passive damper in the driver's seat of a heavy truck.
See ExampleCam Design
Design and prototype mechanisms such as cams with the symbolic component creation capabilities in SystemModeler.
See ExampleHeavy Equipment
Excavator
Explore the reachable positions of an excavator's bucket and visualize the movement.
See ExampleActive Damping
Minimize vibrations in a diesel engine using a tuned mass damper.
See ExampleHydraulic Motor
Study the effect of changing displacement in a hydraulic motor.
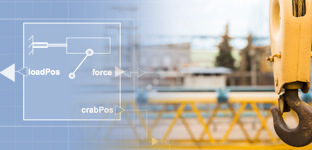
See ExampleModel Inversion Control of a Hoist Crab
In this example, a model of a hoist crab is used to demonstrate the application of model inversion control in controlling nonlinear systems.
See ExampleIndustrial Manufacturing
Model new manufacturing processes prior to implementation or existing ones in order to diagnose problems. Use Mathematica for analyses including pre- and post-processing of models, simulations, and results.
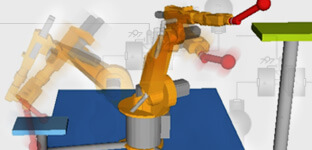
Industrial Robot
Find the optimal way between two points for an industrial robot arm.
See ExamplePrinting Press
Analyze configurations in which bouncing of the cylinders occurs in a flexographic printing press.
See ExampleContinuously Stirred Tank Reactor
Find an optimal control signal for a continuously stirred tank reactor (CSTR), while obeying safety limitations in temperature.
See ExampleStewart Platform
Analyze the movement of a parallel manipulator and visualize its path in 3D.
See ExampleConsumer Products
Design home appliances and other electronics systems with SystemModeler and easily incorporate non-electronic elements, such as mechanical assemblies and temperature control, into the complete design.
Joystick Design
Compare three designs for a spring-based recentering mechanism in an analog joystick.
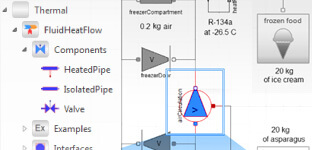
See ExampleRefrigerator Freezer
Simulate a household refrigerator freezer in several scenarios, including handling events such as the sudden triggering of a thermostat.
See ExampleElectric Kettle
Simulate a controlled heating process in a water heater. Combine components from multiple domains and identify parameters.
See ExampleEnergy
Use SystemModeler to develop accurate models of wind, solar, and other power generation and distribution systems. Combine with Mathematica for further optimization and analysis.
House Heating
Compare energy consumption for house heating needs in different locations using measurement data.
See ExampleBattery
This model allows you to analyze how a battery responds to thermal conditions as well as to charge and discharge cycles.
See ExampleLife Sciences
Use SystemModeler for modeling and analysis throughout drug discovery, development, clinical trials, and manufacturing. The flexible environment supports application areas such as systems biology, bioinformatics, and more.
Meal Simulation
Simulates the process that maintains healthy blood glucose levels in the human body after eating a meal.
See ExampleInsulin Signaling
Model a biochemical process and study how insulin signaling in fat cells works.
See ExampleEstimating Liver Function
Create a pharmacokinetic model to describe the distribution and transfer rates of contrast agent between different parts of the body.
See ExampleAn Influenza Epidemic
Model the spread of an influenza virus in a population with components from the SystemDynamics library.
See ExampleBusiness
Enhance managerial understanding by using Wolfram System Modeler to simulate dynamic models of strategic business, economics and public policy issues.
Production Chain
Model a production chain for a company producing a durable good.
See ExampleEmployee Management
Vary the control parameters that relate to aspects of the human resource planning (HRP) process to test different HRP strategies.
See ExampleModeling the World
Combine system dynamics methodology with data from Wolfram|Alpha.
See ExampleOther
Use examples from a wide range of application areas to learn about modeling and simulation with SystemModeler and the Modelica language.
Ice Pod
Model ice contact with propellers and investigate these forces with standardized load test cases.
See ExampleSystem Integration with FMI
Use the Functional Mock-up Interface (FMI) to export a model from SystemModeler and import it in NI VeriStand to test a system in real time.
See ExampleElectrical Circuit Reliability
Use SystemModeler's reliability capabilities to study how heat affects the mean time to failure for an electrical circuit.
See Example