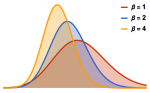
Random Matrices
Mathematica Version 11 introduces support for random matrices. The efficient generation of matrix variates, estimation of their properties, and computations of their limiting distributions are tightly integrated with the existing probability & statistics framework. Random matrices have uses in a surprising variety of fields, including statistics, physics, pure mathematics, biology, and finance, among others.
Key Features
|
|