- Introduce geometric regions as first-class citizens in the Wolfram
Language.
- Full support for a large number of basic and common special regions. »
- Full support for formula regions either implicit or explicit
(parametric). »
- Full support for mesh-based regions in 1D, 2D, and 3D. »
- Full support for derived regions such as Boolean combinations
and transformations. »
- Support for computing measure (length, area, volume, etc.) for
any region, (approximately, exactly, and with parameters).
- Support for computing centroids for any region (approximately,
exactly, and with parameters).
- Support for computing nearest points, distance, and signed distance
from a point to any region (approximately, exactly, and with
parameters).
- Support for testing membership and conditions for membership
for any region.
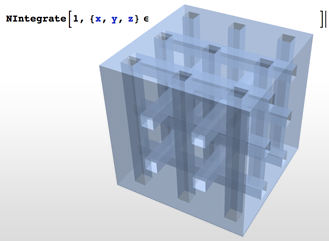
- Support for integration over any region (approximately, exactly,
and with parameters).
|
- Support for solving partial differential equations and systems
of partial differential equations over regions using finite elements.
- Support for optimization using region constraints (approximately
and exactly).
- Support for solving equations (approximately and exactly), inequalities,
and quantifiers (exactly and with parameters) using region constraints.
- Support for regions of any geometric dimension less than the
embedding dimension, e.g. points, curves, and surfaces in 3D
or regions including mixed-dimension elements.
- Support for non-manifold regions. In fact, manifolds are never
a requirement.
- Support for special and formula regions in any dimension.
- Automatic region construction from point sets, including Delaunay
triangulated meshes, Voronoi tessellated meshes, and convex hulls.
- Automatic discretization of 2D and 3D graphics to mesh-based
regions.
- Automatic discretization of any 1D, 2D, and 3D embedded region
to mesh-based regions.
- Automatic triangulation of mesh-based regions in 1D, 2D, and
3D.
|